Optimizing with Quantum Computing: A Revolution Unleashed
Quantum computing, a cutting-edge technology harnessing the principles of quantum mechanics, is poised to revolutionize the realm of optimization problems. From complex logistical challenges to intricate mathematical conundrums, quantum computing holds the promise of unlocking unprecedented solutions.
Understanding Quantum Computing Basics
At the heart of quantum computing lies the qubit, the fundamental unit of quantum information. Unlike classical bits that exist in a state of 0 or 1, qubits can exist in multiple states simultaneously, thanks to the principle of superposition. This unique property allows quantum computers to process vast amounts of information in parallel, exponentially increasing their computational power.
Optimization Problems in Focus
Optimization problems, prevalent in various industries, involve finding the best solution among a set of possible solutions. Classical computers often struggle with the complexity of these problems, especially as the size and variables increase. Quantum computing, with its ability to explore multiple solutions concurrently, presents a game-changing approach to tackling optimization challenges.
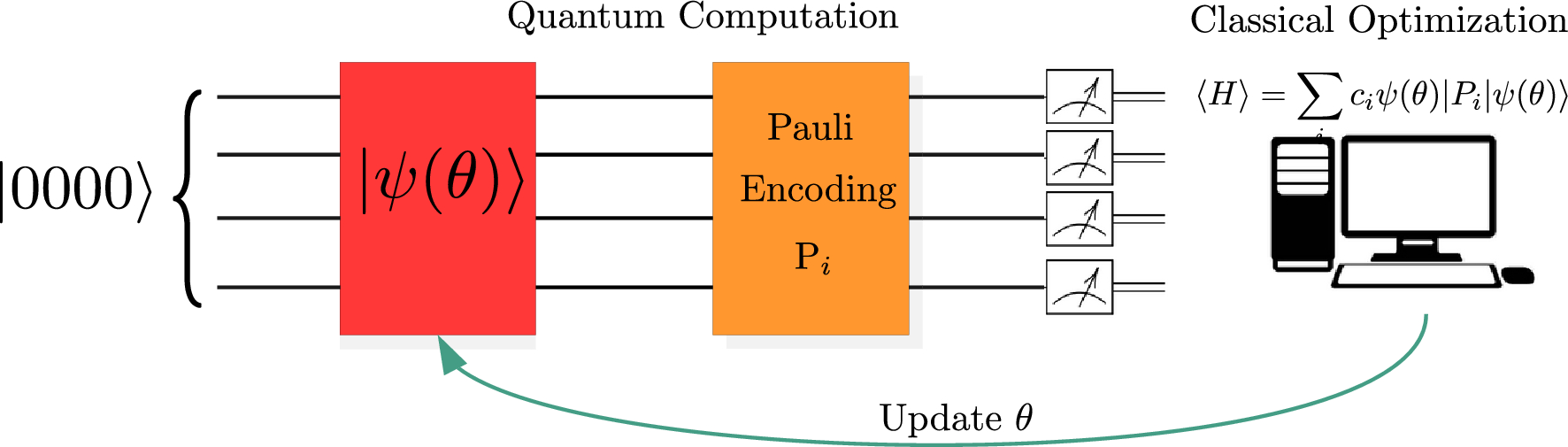
Quantum Algorithms for Optimization
Several quantum algorithms are specifically designed to address optimization problems efficiently. One notable example is the Quantum Approximate Optimization Algorithm (QAOA), which leverages the principles of adiabatic quantum computing to find near-optimal solutions. These algorithms hold the potential to outperform classical optimization methods for certain problem sets.
Logistics and Supply Chain Optimization
In industries reliant on intricate logistics and supply chain management, quantum computing for optimization problems offers a boon. Quantum algorithms can analyze vast datasets and variables, optimizing routes, warehouse placements, and distribution strategies. The result is increased efficiency, reduced costs, and improved overall supply chain performance.
Linking Quantum Computing for Optimization Problems to Real-World Solutions
For businesses seeking to harness the power of quantum computing for optimization, expert guidance is crucial. If you’re exploring the integration of quantum computing into your optimization strategies, visit Quantum computing for optimization problems for insights and assistance. Discover how quantum solutions can be tailored to meet the specific needs of your industry and optimization challenges.
Financial Portfolio Optimization
In the realm of finance, quantum computing holds immense potential for optimizing investment portfolios. The complex calculations involved in assessing risk, return, and portfolio diversification can be addressed more efficiently with quantum algorithms. As a result, financial institutions can make more informed and strategic investment decisions.
Drug Discovery and Molecular Optimization
The field of pharmaceuticals benefits significantly from quantum computing’s optimization prowess. Quantum algorithms can analyze molecular structures and interactions, expediting the drug discovery process. This acceleration in molecular optimization holds the potential to bring new medications to market more quickly, addressing critical healthcare needs.
Challenges and Future Prospects
Despite the exciting potential of quantum computing for optimization problems, challenges such as error correction, hardware stability, and the need for specialized expertise remain. Ongoing research and development efforts are underway to overcome these hurdles. Looking ahead, the evolution of quantum computing technologies promises even more refined solutions and broader applicability.
Conclusion: A New Era in Optimization
In conclusion, quantum computing for optimization problems heralds a new era in problem-solving. From logistics and finance to healthcare and beyond, the impact of quantum solutions is profound. As the technology matures and becomes more accessible, businesses and industries stand to benefit from the transformative power of quantum computing in optimizing complex scenarios.
